Oh, the depth of the riches of the wisdom
and knowledge of God!
How unsearchable his judgments,
and his paths beyond tracing out!
Romans 1:33
“Bottomless wonders spring from simple rules, which are repeated without end… The Mandelbrot set is the most complex mathematical object known to mankind.”
– Benoit B. Mandelbrot
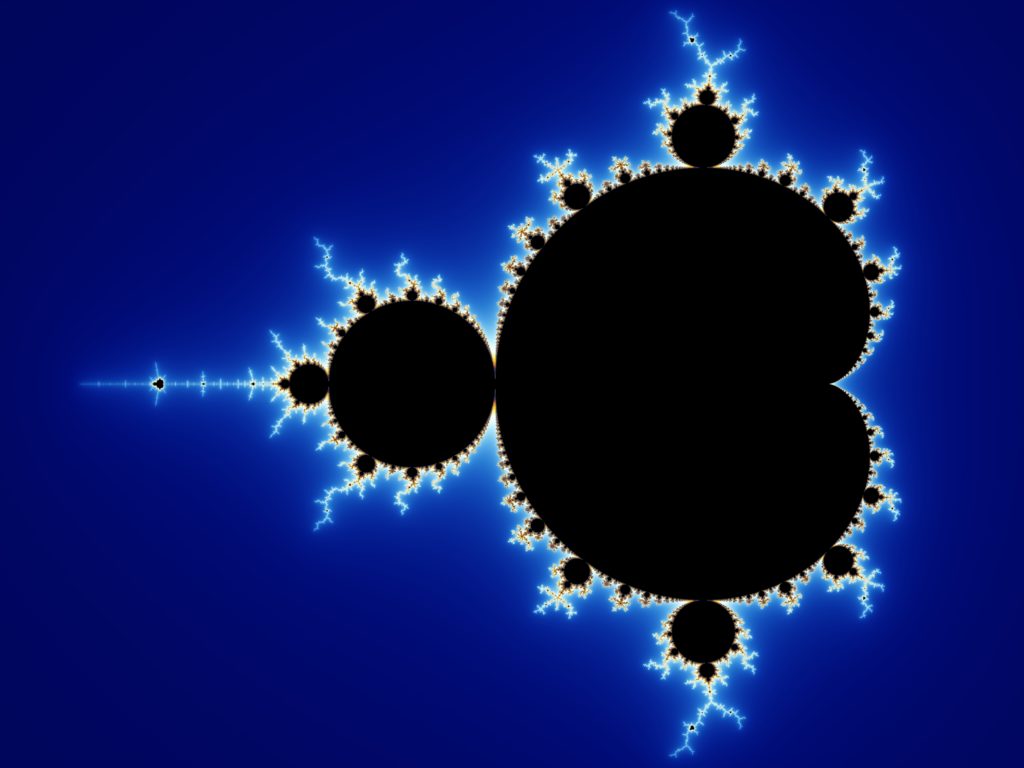
One of the most captivating wonders in the realm of mathematics is the Mandelbrot set. Named after the mathematician Benoit Mandelbrot, the Mandelbrot set is a collection of complex numbers which, when applied to a simple iterative equation, remain bounded in value. The equation used to generate it is z(n+1) = z(n)2 + c, where z and c are complex numbers, n is the iteration number, and z(0) = 0.
It was only in 1978 when computers were powerful enough to be able to plot the equation that people could see the astounding fractal shape generated by the Mandelbrot set equation and were shocked by its unique beauty and infinite complexity.
When plotted on a complex plane, the set produces a visually stunning and infinitely intricate fractal structure. A unique attribute of the Mandelbrot set is its self-similarity; as you zoom into the shape, you’ll see smaller versions of the whole set unceasingly appearing again and again. However, the patterns you find at different scales are only similar, not the same.
The Mandelbrot set contains infinite variety and it never repeats itself as you zoom in, with patterns emerging that sometimes look like a beautiful woven design, other times like a kaleidoscope and other times like the pattern on a seashell. It is for this reason that the Mandelbrot set is often referred to as the “thumbprint of God”. This fascinating blend of simplicity, beauty and complexity is seen by many as a reflection of God’s creativity and intricate design evident in the universe.

Leave a Reply